Sandbox

<math>P \left({A_1 \cup A_2}\right) = P\left({A_1}\right) + P\left({A_2}\right) -P \left({A_1 \cap A_2}\right)</math>

Accidental insights

My collective understanding of Power Laws would fit beneath the shallow end of the long tail. Curiosity, however, easily fills the fat end. I long have been intrigued by the concept and the surprisingly common appearance of power laws in varied natural, social and organizational dynamics. But, am I just seeing a statistical novelty or is there meaning and utility in Power Law relationships? Here’s a case in point.

While carrying a pair of 10 lb. hand weights one, by chance, slipped from my grasp and fell onto a piece of ceramic tile I had left on the carpeted floor. The fractured tile was inconsequential, meant for the trash.

As I stared, slightly annoyed, at the mess, a favorite maxim of the Greek philosopher, Epictetus, came to mind: “On the occasion of every accident that befalls you, turn to yourself and ask what power you have to put it to use.” Could this array of large and small polygons form a Power Law? With curiosity piqued, I collected all the fragments and measured the area of each piece.

Piece	Sq. Inches	% of Total
1	43.25	31.9%
2	35.25	26.0%
3	23.25	17.2%
4	14.10	10.4%
5	7.10	5.2%
6	4.70	3.5%
7	3.60	2.7%
8	3.03	2.2%
9	0.66	0.5%
10	0.61	0.5%

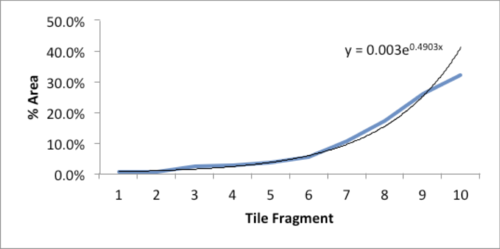
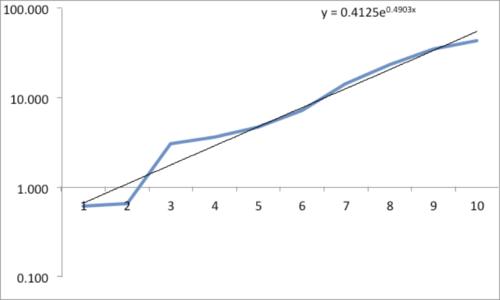
The data and plot look like a Power Law distribution. The first plot is an exponential fit of percent total area. The second plot is same data on a log normal format. Clue: Ok, data fits a straight line. I found myself again in the shallow end of the knowledge curve. Does the data reflect a Power Law or something else, and if it does what does it reflect? What insights can I gain from this accident? Favorite maxims of Epictetus and Pasteur echoed in my head: “On the occasion of every accident that befalls you, remember to turn to yourself and inquire what power you have to turn it to use” and “Chance favors only the prepared mind.”

My “prepared” mind searched for answers, leading me down varied learning paths. Tapping the power of networks, I dropped a note to Chance News editor Bill Peterson. His quick web search surfaced a story from Nature News on research by Hans Herrmann, et. al. Shattered eggs reveal secrets of explosions. As described there, researchers have found power-law relationships for the fragments produced by shattering a pane of glass or breaking a solid object, such as a stone. Seems there is a science underpinning how things break and explode; potentially useful in Forensic reconstructions. Bill also provided a link to a vignette from CRAN describing a maximum likelihood procedure for fitting a Power Law relationship. I am now learning my way through that.

Submitted by William Montante

The hot hand, revisited

Ethan Brown sent the following links to the Isolated Statisticians e-mail list:

• Does the 'hot hand' exist in basketball?, by Ben Cohen, Wall Street Journal, 27 February 2014

• The hot hand: A new approach to an old “fallacy”, by Andrew Bocskocsky, John Ezekowitz, and Carolyn Stein, MIT Sloan Spots Analytics Conference, 28 February-1 March, 2014

The WSJ story is reporting on a new study presented at the MIT conference. The researchers found a "small yet significant hot-hand effect" after examining a data set consisting of some 83,000 shots from last year's NBA games. The analysis was facilitated by the extensive video records now available. In particular, the model took into account the potential increase of difficulty of successive shots in a streak. For example, "hot" players might attempt longer shots, or draw tighter coverage from defenders. Conditioning on the difficulty of the current shot, the researchers found a 1.4 to 2.8 percentage point improvement in success rate after several previous shots had been made.